Connectivity/mathematics learning
Exploiting Connectivity for Mathematics Learning, panel, ICMI 17, HanoÃŊ
 Presentation
Presentation
A Study Conference of the seventeenth ICMI study will take place 3-8 December 2006 and will be hosted by the Hanoi Institute of Technology, Vietnam. The Discussion Document for the Study can be downloaded from the webpage.
The International Programme Committee has decided to have various plenary events at the meeting, one of which will be a plenary panel allocated two hours, titled Exploiting Connectivity for Mathematics Learning. We feel that this is a hugely important topic for consideration by the mathematics community, not least because of our commitment to consider seriously issues of access and diversity. Our idea is that some of the panel participants will be able to be present in Vietnam to make a input on the potential and constraints of connectivity to promote mathematics learning; while others not able to attend in person will present their input either through video conferencing or the internet depending on practical considerations in Vietnam.
Our plan is that after the panellists present their specific vision and experience there will be an opportunity for interaction both between panellists and with conference delegates. Celia Hoyles will chair the panel and, if necessary, introduce issues derived from her own experience and research.
Panelists
Ivan Kalas, Comenius University, Bratislava, Slovakia
Richard Noss, London Knowledge Lab, Kaleaidoscope European Network
Luc Trouche, INRP, France
Uri Wilensky, North Western University, Chicago, USA
Forum preparing the panel
Ivan Kalas
Comenius University, Bratislava, Kaleidoscope European Network
Some issues
In our department we have rich experience in developing interfaces for learning, such as Super Logo, Thomas the Clown, Imagine Logo and others. In the process of their development we try to create effective opportunities for communication and working in common learning spaces. We tend to interpret such spaces differently, from physically common space at one place (like an interactive smart board), to shared space in a classroom, to virtual space shared over the network.
Main vision in our recently completed CoLabs project was to examine obstacles, which obstruct collaborative learning, see e.g. (Turcsanyi/Szabo & Kalas, 2005). In the project we have used our Imagine Logo platform for developing and exploring collaborative microworlds – we call them collaboratories – which would allow children to communicate and cooperate – either locally at one place or through the network in one school or town, or even among different countries – in spite of many (a) technical, (b) linguistic and (c) cultural obstacles.
As one such collaboratory we have created Visual Fractions – a complex dynamic interactive computer environment, which allows groups of children to explore and discover fractions and fractional relations. Visual Fractions provide powerful dynamic jigsaw puzzle pieces for building children's own understanding of the topic, see (Lehotska & Kalas, 2005). One of our research questions was to examine which features of the underlying software platform make effective collaboration possible. However, while evaluating Visual Fractions environment with a group of future teachers we have been pleased to observe that building and exploring fraction activities requires and develops the same skills as programming, see (Lehotska 2006).
Although we were rather successful in the project in getting over all obstacles (technical, linguistic and cultural) mentioned above, we still feel like we are facing yet another one, the biggest of all: (d) educational obstacle. It seems that our formal school systems aren’t quite ready to assimilate computational support for effective collaboration so far.
Present development
Since then we concentrate mainly on how to provide support for collaboration within Imagine Logo environment. Our ambition is to explore equipment that should be offered to teachers, researchers and enthusiastic amateur developers who feel need for small, immersive, open, interactive, flexible and collaborative microworlds developed for everyday learning situations.
When we examine the development of Imagine Logo microworlds from the collaboration point of view, we distinguish four dimensions of this process, namely (1) Technicalities, (2) Connection interface, (3) Sharing and (4) Activity itself (for details about these dimensions see e.g. Kalas & Winczer, 2006). We are particularly interested in building proper metaphors which would mediate computational support for collaboration to teachers and learners in the most intuitive and inspiring ways.
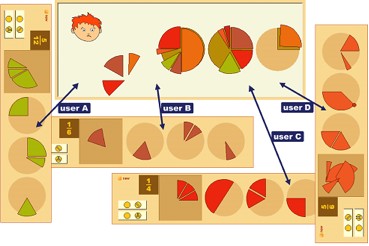
The figure above illustrates an experimental microworld in which several connected users own their personal technical panels, where they have been given several visually represented parts of a whole (fractions). Beside this, all users share a common work space in which they are expected to piece together a given quantity – expressed in shaded circles as an improper fraction, in our example 2¼. Users can bring their own pieces into the common space or manipulate pieces of others (comparing them, rearranging or rotating them etc.) – however, only our own pieces can be dragged back to our personal technical panel, to be „weighted“ there or divided into smaller pieces and then reused in the common space.
Similar approach to collaboration in many possible variations can be innovatively employed with children from preschool to upper secondary stages, in mathematics learning, language skills building, art and design, science, citizenship, social and health education... – through constructing common spaces, comparing, combining and distinguishing various actors and objects, in many different situations where children can compose together, explore, construct, communicate, present or solve problems.
Some questions
I will take advantage of writing these paragraphs after Richard Noss and start by paraphrasing his question: Can we build computational support for effective collaboration? by posing its sophisticated variant: If we build computational support for effective collaboration, will effective collaboration happen?
To put it more straightforward:
- What are the issues that hinder effective collaboration?
and more focused on our own team’s work:
- What are the properties of a software platform, which support and disburden the development of microworlds for effective collaboration?
- Is it possible to build a detailed map of all aspects important for developing collaborative microworlds – a kind of a framework for their development? In (Kalas & Winczer, 2006) we present our first attempt to build such framework.
References
Lehotska, D. & Kalas, I. (2005) LVF – Interface for Dynamic Fractions. Proc. of the 7th International Conference on Technology in Mathematics Teaching, Vol. 1, Bristol: University of Bristol, 2005 pp. 108-116
Turcsanyi-Szabo, M. & Kalas, I. (2005) Collaboration – a tool for learning. Proc. of 10th European Logo Conference EuroLogo 2005, Warsaw, pp. 54-65
Kalas, I. & Winczer, M. (2006) Building interfaces for on-line collaborative learning. To appear in Int Journal Education and Information Technologies, Springer
Lehotska, D. (2006) Visual fractions in teacher training. In Imagining the future for ICT and education: Abstracts and proceedings from the WG 3.1 & 3.5 Joint Conference. Alesund University College, Norway 2006
 Richard Noss
Richard Noss
Some issues
We probably agree among ourselves, at least, that finding ways for students to construct their own mathematical models is a powerful way to foster mathematical learning. Numerous studies have also attempted to exploit the potential benefits of collaborative learning, to encourage – for example - the externalisation and articulation of structural invariants. However, despite some successes, difficulties remain, and these tend to coalesce around the need for intensive, timely and appropriate pedagogic support from the teacher. In particular, I'd like to raise three problematic issues that arise naturally:
i. the need to provide students with appropriate pedagogic support during the modelling process
ii. the need to support students in the analysis of their models and in generalising from particular cases, and
iii. the need to support the building and sustaining of a collaborative community in which interaction is effectively focused on specified learning outcomes.
An illustrative and only partially successful example
There is a particular challenge in designing for collaborative learning in mathematics. To a large degree, this stems from the difficulty of identifying engaging things for students to talk about: mathematical abstractions are not necessarily intrinsically motivating! In our own work, we have addressed this issue by focusing activity on the construction of mathematical objects, for example in the context of six to eight-year old students designing, implementing and sharing videogames (the Playground project see e.g. Hoyles et al. 2002), and more recently, older students exploring the possibilities of building and sharing constructed virtual mathematical objects and relationships (the WebLabs project: see, Simpson et al. 2005; Simpson et al, in press; Noss & Hoyles, 2006; Mor & Noss, in press). WebLabs involved students in building models of mathematical phenomena, although in a very different setting (out of school clubs) from that which we now propose, and without intelligent support. Crucially, we constructed a virtual collaborative space, WebReports, in which we tried to encourage students to share their models, and collaboratively extend their knowledge through asynchronous peer interaction (see the website). Two findings stand out. First, certain kinds of activities and tasks, based on competitive game-like scenarios, achieved relatively high levels of successful interaction. Second, we achieved rather little success in effective knowledge building, the modal thread length of an interaction being only 1. Scardamalia and Bereiter report much the same, (Scardamalia & Bereiter, 2006). Moreover, successful cumulative knowledge construction was critically dependent on teacher intervention directly to facilitate, encourage and foster interactions (for example, to draw attention to conflicting reports posted or to interesting extensions or different representations of the problem).
We found that support of this kind was essential, but massively labour intensive. WebReports was, in effect, nothing more than a database with a relatively friendly interface with intelligent intervention resided with the teacher or researcher. This work has forced us to recognise the need to build intelligent support, not only into the process of model construction by an individual (the eGeneraliser), but by designing intelligent support for effective (i.e. productive) collaboration and the development of a sustainable community where interaction and collaborative knowledge building becomes the norm.
So, a question
Can we build computational support for effective collaboration?
Some references
Hoyles, C., Noss, R. and Adamson, R., (2002) Rethinking the Microworld Idea. Journal of Educational Computing Research, 27(1&2) pp. 29-53
Mor, Y. & Noss, R. (in press) Programming as mathematical narrative International Journal of Continuing Engineering Education and Learning
Mor, Y. Noss, R. Hoyles, C., Kahn K. & Simpson G. (2006) Designing to see and share structure in number sequences. Submitted to: International Journal for Technology in Mathematics Education. 13 (2), 1-14.
Noss R. & Hoyles, C. (2006) Exploring mathematics through construction and collaboration. In: Sawyer, K. (Ed) Cambridge Handbook of the Learning Sciences. Cambridge UK: C.U.P. 389-408.
Simpson, G., Hoyles C. & Noss R. (2005) Designing a programming-based approach for modelling scientific phenomena. Journal of Computer Assisted Learning. 21, pp. 143–158.
Simpson, G., Hoyles, C. & Noss, R. (2006) Exploring the mathematics of motion through construction and collaboration. Journal of Computer Assisted Learning. 22, pp.1-23
 Luc Trouche
Luc Trouche
Questions
What are the potentialities and constraints of a new environment aiming at facilitating connectivity between students? What are the effects on the learning processes and the students' behaviour? What are the new difficuties and opportunities for the teacher?
From a theoretical point of view, does connectivity raise some new challenges for the ideas of webbing and orchestrations?
Illustration
To introduce the discussion on these questions, it is necessary to analyze precisely what happens in a classroom when a new technology is introduced, connecting students and teachers. I have asked Laurent Hivon, the head of the team experimenting TI-Navigator, to describe this research project, its hypothesis, the first results... and some new questions.
CROME Project, presentation from Laurent Hivon
Network Calculators : Orchestrations and Mutualisations in a new Environment
Working with INRP, IREM and IUFM, a team of six high school teachers (students aged from 15 to18) living near Orleans (France) are studying how to introduce and work with the TI Navigator System in a classroom. This system provides wireless communication between students' TI graphing calculators and the teacher's PC. This system is not yet available in France, but it is tested in some other countries (USA, CANADA for example). This teachers' team, working with this system since November 2005, is going to carry on this project until at least 2007. The following paragraphs allow to learn more about this project.
1. Description
The research hypothesis of the team is that the integration of this new system in a classroom could deeply change the mathematical situations proposed to the students and could make the teacher change the specific orchestrations. The implementation of this device could also change the relationships between pupil and class by giving rise to debates among peers. This system allows the teacher to give his/her students a lot of new open or closed exercises, to make quick polls or to gather into a single file the data of all the students' calculators (when working on statistics for example).
2. First results
Instrumentalization processes
In the very first use of the Navigator System, we observed instrumentalization processes, especially when students worked on the same public worksheet. Here are some examples:
- when they had to draw points on the public screen, some students tried "to catch" each other's points,
- when they had to draw a curve, they tried to copy somebody else's curve, they tried to invent the most difficult curve they could.
"Return" actions
Students' behaviour seems to be deeply modified by public display (anonymous or not) of their work. But, in spite of the fact that French students are often deaf when teachers ask them to create mathematics objects, this system seems to make them freer to display what they created. Knowing that everyone has to produce something everyone can see, seems to set them free to create mathematics. In a traditional system, the behaviour of a student mostly depends on the teacher's. In a system relying on a strong connectivity, the students' behaviours interact on each other.
For example, displaying the calculators screens -Screen Capture - produces several effects on the students:
- the first displayed products help the other students to go ahead,
- as the responses proposed by the students are often very different, each one can use a lot of ways to find an answer, and not only one as in a traditional course,
- they often try to produce the most complicated object they can.
In the future, when students get used to working with this system, we will have to try to know if most of them do not wait for the first answer and just copy it...
How to observe the students' work?
It seems hard to observe the students' work because of three major reasons:
- complexity of the orchestrations: as a lesson is made up of many stages (personal work, mutualisation inside each group, mutualisation in the whole classroom, debates), it is not so easy to observe the way a student changes his/her mind;
- many instruments are involved in the students' work: as the students use paper, screen calculator, public screens..., it is more difficult to know what s/he does and in which order s/he does it;
- the way the class is organized: as the students work in groups of four, we can classify their work in the classroom in three involved stages: first, they express their personal point of view (especially at the beginning of the lesson); secondly, they express themselves as members of their group; thirdly, they express themselves as members of the class. Of course, the three ways are mixed.
3. A few questions
Here are a few questions which could be explored in the future.
Teacher's role in the classroom
− the teacher's behaviour is deeply modified. He often appears as a person looking at the building of an object. He gives the students the conditions to build a mathematical object. But this object will be built by the community of the students. A student does not play only the music written by the conductor anymore. He is writing part of the music. The question is now to know how the teacher can create the conditions to make the music not too different from what he wants it to be!
- a recent report of the European Commission shows that French teachers "do not use computers and the internet very frequently and intensively in schools". Do connectivity tools like Navigator could make this situation change?
Public display in the classroom
In TI Navigator, public display is a very important part of the connectivity system and a lot of possibilities are available, for example:
- displaying anonymously or not,
- when and how often the calculators screens of the students can be displayed,
- displaying or not during research stages,
- which screens and which results can be displayed to the students?
- displaying or not the mathematical expressions of the displayed objects (coordinates, curves expressions...).
Knowledge construction and connectivity
In a situation of connectivity, a student constructs her/his knowledge in collaboration with the other students. So, as everyone takes part in this construction, will those conceptions help him or not to build her/his own object?
- how will they learn to manage this new situation? What influence will it have on the way they build their conception of an object?
- in which way, private practice of connectivity (blogs, chat, MSN...) will have an influence on their way to use connectivity inside the classroom?
- will this private practice of connectivity make the instrumentalization stage more important than in a non-connectivity activity at school?
References
Guin, D., Ruthven, K. and Trouche, L. (eds.): 2004, The didactical challenge of symbolic calculators: turning a computational device into a mathematical instrument, Springer, New York
Hivon L.: 2006, Vers une mutualisation de l’usage de la calculatrice, MathÃĐmaTICE.
Trouche, L.: 2004, 'Managing Complexity of Human/Machine Interactions in Computerized Learning Environments: Guiding Student's Command Process Through Instrumental Orchestrations', International Journal of Computers for Mathematical Learning 9, 281-307.
Uri Wilensky
Questions
Illustration
References










